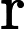PHY385 Module 4: Wavelength of Light

   Activity 4.1 – Young’s Double Slit – Eyeball Method

In two-slit interference, light falls on an opaque screen with two closely spaced, narrow slits. As Huygen’s principle tells us, each slit acts as a new source of light. Since the slits are illuminated by the same wave front, these sources are in phase. Where the wave fronts from the two sources overlap, an interference pattern is formed.

The PASCO OS-8500 optics bench is shown in the figure.  The light source, component holders, and ray table base all attach magnetically to the bench.  For proper alignment, the edge of each of these components should be mounted flush to the alignment rail, which is the raised edge that runs along one side of the bench.

Figure 1.  The setup.  You need to put your head close to the optics bench on the right and look through the diffraction plate towards the light source in order to see the diffraction pattern.

 

The Slit Mask should be centred on the Component Holder. While looking through the Slit Mask, adjust the position of the Diffraction Scale so you can see the filament of the Light Source through the slot in the Diffraction Scale.

Attach the Diffraction Plate to the other side of the Component Holder, as shown. Centre pattern D, with the slits vertical, in the aperture of the Slit Mask. Look through the slits. By centring your eye so that you look through both the slits and the window of the Diffraction Plate, you should be able to see clearly both the interference pattern and the illuminated scale on the Diffraction Scale.

NOTE: In this experiment, you look through the narrow slits at the light source, and the diffraction pattern is formed directly on the retina of your eye. You then see this diffraction pattern superimposed on your view of the illuminated diffraction scale. The geometry is therefore slightly more complicated than it would be if the pattern were projected onto a screen, as in most textbook examples. (A very strong light source, such as a laser, is required in order to project a sharp image of a diffraction pattern onto a screen.)

Figure 2. The patterns on the PASCO “Diffraction Plate”.  For pattern D, the slit spacing for the double slit is 0.125 mm.

 

The essential geometry of the experiment is shown in Figure 3. At the zeroth maxima, light rays from slits A and B have travelled the same distance from the slits to your eye, so they are in phase and interfere constructively on your retina. At the first order maxima (to the left of the viewer) light from slit B has travelled one wavelength farther than light from slit A, so the rays are again in phase, and constructive interference occurs at this position as well.

 

Figure 3. Geometry of two-slit interference.

 

At the n^th order maxima, the light from slit B has travelled n wavelengths farther than the light from slit A, so again, constructive interference occurs. In the diagram, the line AC is constructed perpendicular to the line PB. Since the slits are very close together (in the experiment, not the diagram), lines AP and BP are nearly parallel. Therefore, to a very close approximation, AP = CP. This means that, for constructive interference to occur at P, it must be true that BC = nλ.

From right triangle ACB, it can be seen that BC = AB sin θ, where AB is the distance between the two slits on the Diffraction Plate. Therefore, AB sin θ = nλ. (The spacing between the slits, AB, is listed in Figure 2.) Therefore, you need only measure the value of θ for a particular value of n to determine the wavelength of light.

To measure θ, notice that the dotted lines in the illustration show a projection of the interference pattern onto the Diffraction Scale (as it appears when looking through the slits). Notice that θ´ = arctan X/L. It can also be shown from the diagram that, if BP is parallel to AP as we have already assumed, then θ´ = θ. Therefore, θ = arctan X/L; and AB sin (arctan X/L) = nλ.

 

Figure 4.  This is a drawing of approximately what you are supposed to see.  It is difficult, but with one eye you should be able to see both the diffraction scale and the diffraction pattern at the same time, one just above the other. The zeroth order central maximum should always appear exactly at the 0 cm mark on the diffraction scale.  In this drawing, it appears that the n = 4 order is a distance of X = 9 mm away from the central n = 0 maximum.

 

Looking through the pair of slits (pattern D) at the Light Source filament, make measurements and make two tables like the one below (one for the red filter, one for the green filter).  All of the team members should try this and record individual measurements of the apparent distance between two bright fringes (X) and the number of dark fringes between them (Δn).  In this way you can make four independent determinations of λ.  The error in the mean is \(\sigma/\sqrt{4}\) , where σ is the standard deviation of the four measurements.  Perform the calculations shown to determine the central wavelength of Red and Green light.

 

          Filter: (Red or Green)

Double-slit pattern D: state value of AB

Distance between slit-pattern and the diffraction scale: L =                                           .

Measurement #	Δn	X	\(\lambda = \left( {AB \over n} \right) \sin[\arctan(X/L)]\)
1
2
3
4
Average λ
Error in λ

 

 

 

 

    Activity 4.2 – Young’s Double Slit – Viewing Screen Method

In two-slit interference, light falls on an opaque screen with two closely spaced, narrow slits. Lasers are a good source of very bright, monochromatic, coherent plane waves.  If the laser beam evenly illuminates both slits, you should be able to see the pattern diffusely reflected on a viewing screen.  In this case, the geometry is much easier to visualize than it is when you use your eye as the detector.  See Hecht Section 9.3.1, particularly the geometry shown in  Figure 9.8 on page 394.  A simpler diagram using the same symbols is given below.  Hecht derives the fringe spacing as Eq. 9.30:

\(\begin{eqnarray*} \Delta y = {s \over a} \lambda \end{eqnarray*}\)

Use the red, green and blue lasers and the double slit pattern D to form fringe patterns on the viewing screen.  Count the number of fringes in some known distance to obtain a measurement of Δy.  Measure s, and use a = 0.125 mm to determine λ for each laser.  Repeat the experiment with slit patterns E and F, both of which have a = 0.250 mm.  Not all measurements may be possible; report in your lab book which pattern and choice of s work best for each laser.

 

    Activity 4.3 – Problem solving

Problems 9.4, 9.5, andn 9.8